
As I said, the important point of the single slit experiment is in the probability - whether the electron hits the slit material or makes it on through to the detector is actually immaterial. But, for the graphically inclined, I include a primitive picture of a single slit apparatus, along with the results:

![]()
![]()
![]()
![]()
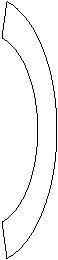

sensor
electron emitter
graph representing a probability distribution: 'hits' at the sensor
electron in flight
slit material
This really is a simple experiment. If the electron makes it through the slit, it registers at the sensor array as a 'hit'. If it instead hits the slit material, well, tough luck. Now, just because the electron is a particle of matter, doesn't mean you can think of it as a tiny solid thingy flying merrily through space. In fact, the electron in flight moves like a wave. In 1924 the physicist Erwin Schrödinger (1887 - 1961) introduced a wave equation in quantum physics, now known as the Schrödinger wave equation, describing the exact nature of this 'matter wave'. Wherever the matter wave comes into contact with an object, whether it hits the slit material, or the sensor, or your finger, the matter wave is said to collapse (people have written very expensive books on this process - on just how the particle makes its tough decision to collapse or not to collapse. But, really, despite all the heavy math, the actual process remains a mystery).
At the point of collapse, it once again takes on the characteristics of a particle - this is where you detect it. All the graph represents is the point of maximum (and minimum) wave collapse if the electron hits the sensor. In my simplistic picture, where everything is in a straight line, the maximum probability will occur in line with the slit -- in other words, the sensor will register more hits in the center than anywhere else, so this is where the graph will peak - this is the point of maximum probability. It is possible to skew the graph and alter the peak probability point by placing the electron emitter on a track and rolling it up or down, parallel to the experiment setup. This will skew the graph, which means the experimenter can play around with the probabilities - an electron might have a 90% chance of passing through the slit under normal circumstances, but by moving the emitter, the experimenter can alter the probability to any percentage he chooses. Why is this so important? As I have said, quantum mechanics is all about probability. But, it is a physics of statistically large numbers of particles. This simple experiment is also about probability - but it concerns the probability of the individual particle. We need to understand individual particles -- the way quantum mechanics is built today, it is almost completely useless when it comes time to deal with single particles. The single slit experiment deals with individual particles, and it is simple enough to understand without getting lost, because it turns out that this 'simple experiment' is like a tiger masquerading as a pussycat. It is important to not get lost.
For example , the wave equation governing the moving electron is a complete, 100% wave equation. There is nothing unambiguous about it. Yet, when we speak about the collapse of this wave function we talk about it in terms of partial percentages - like the way I used the 90% figure when I described the chance of the electron making it through to the sensor when the emitter was placed on a track. This is a very esoteric point: the electron wave is complete, 100%. Then we give the electron a percentage less than 100% to make it through the slit to the sensor. I gave it 90%. I guess you could call this the in-flight statistics. It means the electron has a one in ten chance of hitting the slit. It's also only half the picture - remember, Galileo almost came up with the law of inertia - he knew from his experiments that an object will continue in its state of motion until something else gets in the way. Newton finished off the incomplete law by adding that the object will also continue in its state of REST until acted upon by something... and Newton got most of the credit, and it is one of *his* three laws of motion... poor Galileo. This is a perfect example of a small but significant point. Like I said: esoteric as heck.
When the electron does eventually
get stopped, we say the wave function collapses, forming a complex particle, what everyone else calls a 'real particle',
where this occurs. Since you can't have a portion of an electron only, the probability of finding the electron
where its wave function collapses is 100%. And, this is exactly what we see with
our equipment - a whole electron at some particular point in space. But there is
a tiny problem with this reasoning - the wave function, Schrödinger's equation,
is mathematically imaginary. This is fine for normal quantum mechanics, because
the mathematical operator, i, allows for real quantities we measure, like
position or momentum, to always be real quantities when we solve the equation
(remember from high school algebra that squaring an imaginary operator yields a
real quantity). However, for our purposes, working with the imaginary quantities
directly yields more valuable insights.
There was a 10% chance of the
slit material stopping the electron, and at the same time there was a 90% chance
of the target sensor stopping the electron. Now, both the 90% path and the 10%
path both add up to 100% - no matter how you do this dilly of an experiment, the
mean free paths will always add up to 100%. When the electron's matter wave collapses, this
too, will add up to 100% - there will be a 100% likelihood of finding a whole
electron somewhere - exactly where doesn't really matter.
This is where it ends for most experimenters. The electron matter wave collapses back into a particle which is detected. But is it really all over? Remember, we are dealing with an imaginary wave. Is the 100% probability representing the wave collapse the same 100% (90% + 10%) probability representing the flight path? If it is, then it really is the end of the story. If it isn't, then things get complicated for even this, the most simple of quantum experiments. How so?
When a wave function representing any particle, not just an electron, collapses, it will always be 100%, which is the same as saying you saw a detected event. This can only happen if there is a100% likelihood that there was a collapse - or a hit on the sensor, for example. But for a particle in flight, there represents only a portion of that 100% because there are two or more possible outcomes of the experiment. In one outcome of our experiment, there was a 10% chance of hitting the slit material, and a 90% chance of making it all the way to the sensor at the target. It is the imaginary part of the wave that determines this. Why? Because when an imaginary wave is on the move, referencing it in terms of real (complex) particles doesn't make sense. 90% of the wave made it through the slit and 10% of the wave did not simultaneously. What causes the wave to collapse where it does? No one knows. But let's say the wave hit the slit and collapsed. Now you have a complex particle at the slit. But what about the other 90% - the portion that made it through? It has no real energy because the particle already collapsed, but just because the particle collapsed on the 10% branch does nothing to affect the other un-collapsed wave fronts. Though I kept it simple, in reality, this 90% actually represents ALL the un-collapsed and now imaginary pathways - quite literally an almost infinite number of unrealized pathways.
This is what I call the true probability wave. It is now a permanently imaginary probability wave. Bereft of real energy, it can not make its effects known in the universe any longer. But there are more points that can be made about them. First, though fully imaginary, they are still probability waves. Second, because they had their origins in the matter wave they came from, they still possess exactly the same characteristics of that wave. Also, they still possess the same level of probability they had when they were created. Simply, the information of the complex wave is the same as the information carried in the imaginary wave. So really, we are talking about two different mechanisms here. One mechanism utilizes complex probability to create detectable events - we call this probability mechanism 1 - P1. The other mechanism provides a methodology that makes use of in-flight (complex or imaginary) probability to create a remnant wave and we call this probability mechanism 2 - P2. I call these imaginary probability waves 'P2 Remnant Waves' because they are the left-overs when matter waves collapse and represent all the un-collapsed branches of your experiment. Why not just complex or just imaginary? Because we don't know at this point in time the mechanism for collapse. The obvious difference is that P2 remnant waves never collapse. They just go on and on.
Something else to ponder: Remember earlier when I stated my belief that we 'discover' nothing unique? Somewhere, somehow, nature is already using everything we stumble upon? This is the case for probability waves, both P1 and P2, as well. Every particle interaction from the beginning of time, naturally creates a plethora of both P1 and P2 waves. Natural interactions that result in a single wave function collapse can give rise to an almost infinite number of P2 branches that never collapse, yet still must exist. Whether naturally occurring or man-made, P1 branches belong to the experiment that creates them, but P2 branches in all their infinitude belong to the entire universe, and perhaps beyond... in short, the universe is chock-full of P2 remnant waves. If you could see them you'd be able to cut through them with a knife! It is not unreasonable to suppose that with something so numerous and easy to produce, the universe has had enough time to figure out what else to use P2 remnant waves for? It turns out P2 waves may be even more important than I originally thought.
Also, I can hear those out there who are saying at this
point that probability waves are already complicated enough. Breaking them apart
into P1 and P2 waves seems messy. Actually, it is messier to not break them up
in the manner I have. Remember, a matter wave has a real and an imaginary part.
Quantum operators encode information on both parts of the wave. When a wave
collapses, the real information becomes the particle we can see and measure.
This takes care of the real side of the equation. What about the imaginary
side of the equation? Does the information encoded within the imaginary wave
simply disappear? Information can't be destroyed. This is a significant point -
why would nature destroy something so useful? It doesn't... an apt
analogy can be found in the Black Hole Information wars... even a black hole
can't destroy information. And, as I have further discovered, Nature does indeed
need the information encoded within P2 remnant waves for many useful things, not
just for allowing us humans to create FTL walkie-talkies... so the universe
would not look the way it does today if this weren't so. An example is the way
energy is allocated between P1 and P2. If P1 and P2 are separate entities
moving along different paths, how does P2 know the wavefunction collapsed at P1?
If it didn't there would be the potential for a single wavefunction to collapse
many times, making a mess of energy conservation at the very least. A way to
think about how the universe avoids this scenario is to consider that P1 and P2
are very strongly self-entangled. The real energy is given to a single branch,
which collapses into a particle, while at the same time there is now no energy
in any of the other branches so they never collapse (unless energy is provided
).
You may want to re-read this section over. Faster Than
Light Communications can't be performed with real waves. If we use remnant
waves, the P2 function can effectively side step the energy limitation and make
a working FTL communicator possible - after all, what does an imaginary speed
mean? The universe once again comes to our rescue. We had to find a way to rid
ourselves of energy, which can only travel at the speed of light, and Nature
Provides. All we have to do is find a way to encode usable information on P2
remnant waves and use them instead of their complex brethren, as we do in
regular AM radio. Remember, information encoded in a complex wave is what
gives a real particle its properties, and the same holds true for imaginary
waves. And, as I said above, the information in both is the same. Actually,
looking at it from this point of view, FTL transmission is very simple
to do - but finding a way to build a P2 receiver took me over twenty
years...well there's more to say in yet to be written pages...